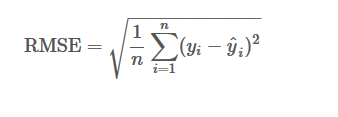Reference Designer Calculators
RMS Error
We will understand Root mean squared error with an example. Assume that a company makes nuts for car company. The inner diameter of the nuts is supposed to be 2.50 mm. However, in production, some have smaller and others have larger dimensions, both of which are bad. The Table below lists the dimensions of the nuts measured in actual practice an error resulted from it.
| Desired Diameter = 2.5 mm |
||||
|---|---|---|---|---|
| S No |
Actual Diameter |
Error |
Mean Absolute Error |
Sqaure |
| 1 | 2.4 |
-0.1 | 0.1 |
.01 |
| 2 | 2.6 | 0.2 | 0.1 |
.01 |
| 3 | 2.1 | -0.4 | 0.4 | .16 |
| 4 | 2.9 | 0.4 | 0.4 | .16 |
| Average Mean Absolute Error = 0.25 |
||||
| Average RMS Error = 0.291548 |
||||
One way to estimate the error is to take the absolute values of the errors and just take average of it. This is fine if the severity of small and big errors are same. But if we wish to penalize bigger errors more, we use root mean square error. In the above example, notice that the RMS error is more that the mean absolute error. If there were only first two rows, the Mean Absolute error and rms error would have been same.
The root mean squared error is defined as