Reference Designer Calculators
RC Time Constant Derivation
The circuit shows a resistor of value $R$ connected with a Capacitor of value $C$.
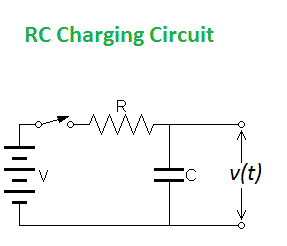
Let a pulse voltage V is applied at time t =0. The current starts flowing through the resistor $R$ and the capacitor starts charging. As a result of this the voltage $v(t)$ on the capacitor $C$ starts rising. The charge $q(t)$ on the capacitor also starts rising. The amount of the charge $q(t)$ at any time $t$ is given by
$q(t) = C.v(t)$
where $C$ is the Capacitance of the capacitor.
Differentiating this equation with respect to time gives
$\frac{dq(t)}{dt} =C.\frac{dv(t)}{dt}$
$\frac{dq(t)}{dt}$ is the rate of the change of charge in the circuit which is nothing but the current flowing. So we can write the equation as
$i(t) =C.\frac{dv(t)}{dt}$
Notice that this current $i(t)$ flows through the resistor
If $v(t)$ is the voltage across the capacitor at time t, then the voltage across the resistor at time t is given by $V-v(t)$.
By Kirchoff's law, we can write
$V-v(t) = i(t). R$
Combining the two equations we get
$\frac{V-v(t)}{R} =C.\frac{dv(t)}{dt}$
Separating the variables.
$\frac{dt}{RC} =\frac{dv(t)}{V-v(t)} $
Solving this equation leads us to
$ \frac{t}{RC}= -ln(V-v(t)) + K$
where K is a constant of integration.
Since, at t =0, v(t) = 0, we get by substitution
$K = ln(V)$
We therefore get
$\frac{t}{RC} = -ln(V-v(t)) + ln(V)$ or
$ ln\frac{V-v(t)}{V} = -\frac{t}{RC}$
Expressing in exponent form, we get
$ V -v(t)= e^{-\frac{t}{RC}} $
which simplifies to
$ V -v(t)= V.e^{-\frac{t}{RC}} $
It is more conveniently expressed as
$ v(t)= ( 1-e^{-\frac{t}{RC}}).V $
when $t = RC$
$v(t)= (1-e^-1).V $
So RC time constant can also be defined at the time at which value of the voltage $v(t)$ reaches to $(1-e^{-1})$ or about 63.2% of final value